|
Cross+A  パズルのバリエーション パズルのバリエーション
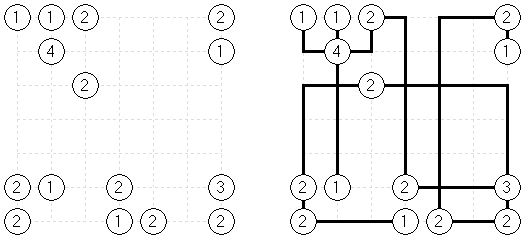
スリザーリンク(Slitherlink) シープ&ウォールブス(Shipu & Woruwusu、Sheep and Wolves)とは、スリザーリンクという種類のパズルの一つである。0から3までの数字以外黒の〇印(シープ)と黒の×印(ウォールブス)は入る。そのパズルが解けた後、「シープ」は閉じた線の内側に、「ウォールブス」は閉じた線の外側になければならない。
バトルシップ(Battleships) ディジタル・バトルシップス(Digital Battleships)は枠には全て数字がある。横列、縦列の網の外の数字はそれぞれのシップの部分の合計を意味する。シップは繋がれない。
Retrograde Battleships (Reverse Battleships) contains all segments of the ships in the grid. The aim is to find the correct locations of the ships. No ship may touch another, even diagonally.
フィルオミノ(Fillomino) 長方形のないフォルイミノ(No Rectangles Fillomino)には追加ルールがある。ブロックは長方形または正方形の形にしてはいけません。
長方形のみのフォルイミノ(Only Rectangles Fillomino)には全てのブロックは長方形または正方形の形になっている。
2×2 正方形のないフォルイミノ(No 2×2 Squares Fillomino)同じの数字が入っている2×2 正方形がないのは追加ルールである。
非連続フォルイミノ(Non-Consecutive Fillomino)には縦・横に隣接するブロックに、数字の差は1になってはいけない。
一つ違いフォルイミノ(Consecutive Fillomino)に各ブロックは、一つ違いの数字が入っている少なくとも1つのブロックに縦・横に接する。
縦・横列の重ならないフォルイミノ(No Row/Column Repeats Fillomino、Deadomino)縦・横列に配置する全ての同じの数は同じのブロックに入いっている。例えば、列には5が2つ入ったら、同じのブロックの5に限られている。
奇数の フォルイミノ(All Odds Fillomino)には全てのブロックの面積は奇数である。
偶数の フォルイミノ(All Evens Fillomino)には全てのブロックの面積は偶数である。
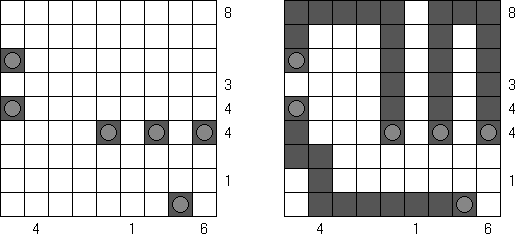
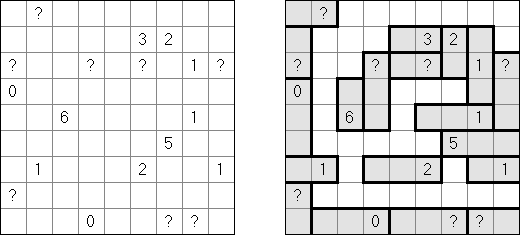
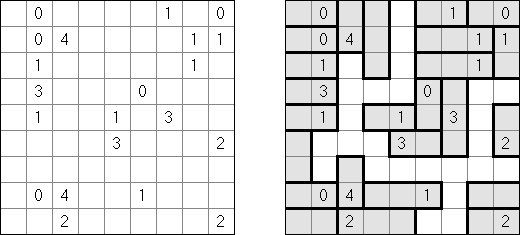
ぬりかべ(Nurikabe) Line Nurikabe: a grid cannot contain five consecutive black cells in a row or column (2 x 2 cell area can be all black).
塗り壁のペア(Pairs Nurikabe)には通常の塗り壁と違って、各島には数字が2つ入る。数字の合計は島の面積を表す。
スカイースクレーパー(Skyscrapers) ビルディングの和(Sum Skyscrapers)は変則ルールのビルディングパズルである。変則ルールは、外側の数字がその列で見えるビルの階数の和を表す、ということである。
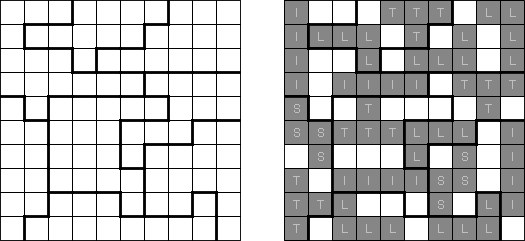
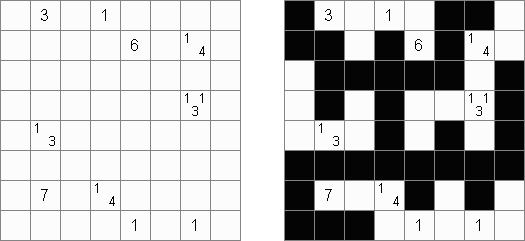
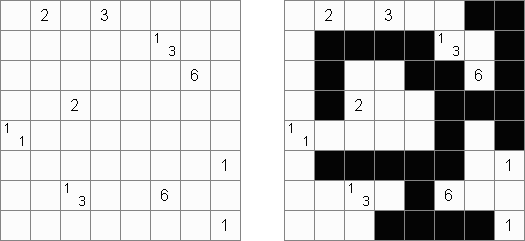
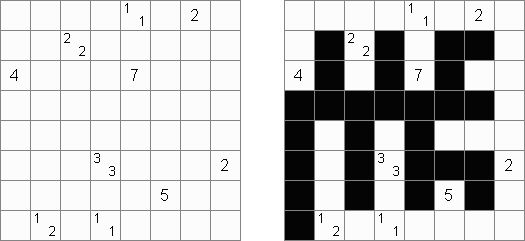
タパ(Tapa) タパパズルのバリエーションがたくさんある。Cross+Aのプログラムは次のようなバリエーションを解ける。 タパライン(Tapa [Line])の変則による、4つの黒マスは縦・横に連続できない。
スクウェアのないタパ(No Squares Tapa)には白マスの 2×2 の固まりを作ってはいけない。
平等なタパ(Equal Tapa)には白マスの合計と黒マスの合計は平等である。数字を含めるマスは白マスではない。
黒白タパ(B&W Tapa):
タパ島(Tapa Islands):
パタ(Pata):
タパバランス(Tapa Balance)には盤面の右側にある黒マスの合計と左側にある黒マスの合計は平等である。白マスとヒント数字を含めるマスを数えない。
タパの列(Tapa Row)には縦・横列の数字の合計は、その列の黒マスの合計を表す。
タパ1-n (Tapa 1-n)にはすべての列の黒マスの合計は異なる。
分割されたタパ(Dissected Tapa)には黒マスと白マスは2つの合同図形を成形する。合同図形はサイズも形も全く同じである。
タパ[斜めの隣接](Tapa [Diagonal Neighbors])には各黒マスは少なくても1つの黒マスに斜めに隣接する。
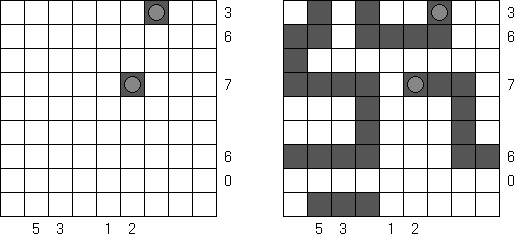
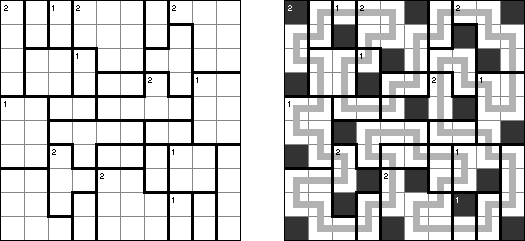
バッグ(Corral) インサイド/アウトサイドバッグ(Inside/Outside Corral)とはバッグパズルの変動の1つである。通常のバッグと違って、盤面の数字は輪の内側だけでなく、外側にも入ることができる。いずれの場合も、ヒント数字は、 その数字から上下左右4方向にまっすぐ進み、輪の線にたどりつくまでの、その数字を含めてのマスの合計を表す。
アルコネ(Arukone) Arukone3:
ABCプレース(Easy as ABC) Not as Easy as ABC is a variation of Easy as ABC puzzle. The goal is to fill in the first letters of the alphabet on every row and every column exactly once. One cell in every row and every column remains empty. Letters and numbers on the outside indicate at what position you come across this letter when looking from that side (e.g. C2 means the C is the second letter encountered when looking from that side).
イチマガ(Ichimaga) 交差もするの(Crossing Ichimaga): the lines may cross other lines; the lines cannot change direction at the point of intersection.
磁石イチマガ(Magnetic Ichimaga): the circles with the same digits cannot be connected.
へび(Snake) Multiple Snakes: a grid contains multiple snakes. Head and tail of all snakes are given. Different snakes do not touch each other, even diagonally.
Toroidal Snake: a grid wraps around itself. A snake can go from one edge to another.
マインスイーパ(Minesweeper) Double Minesweeper: place mines into each empty cell in the grid, at most two mines per cell.
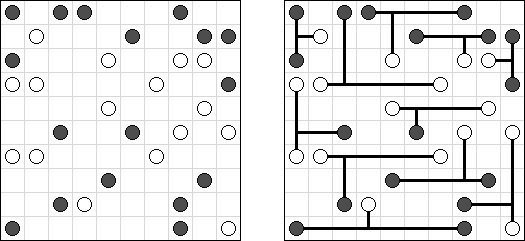
ミルクティー(Milk Tea) Milk Tease is a variation of Milk Tea puzzle. T-shaped line may connect:
Two circles of the same color must be connected by the straight-line segment of the T-shaped line.
美術館(Light Up) Mirror Akari ("!irakAkari!"): a square or rectangular grid contains diagonal walls (black triangles) with mirrors.
パーキング(Tren) Tren+:
Ghost Tren:
ムーブナンバー(Mubunanba) Mubunanba+:
ヤジリン(Yajilin) Regional Yajilin (also known as "Yajilin (Regions)") is a square or rectangular grid divided into regions. The aim is to blacken some cells and to draw a single non-intersecting loop through all the white cells. A number in a region indicates the number of black cells in that region. A region without a number can contain any amount of black cells. No two black cells can share a border. The loop may visit numbered cells; numbered cells can be blackened.
ヌルオミノ(LITS) Double LITS has one difference from the classic variant of the puzzle: each region must contain two tetrominoes. These two tetrominoes within a region cannot touch each other horizontally or vertically (only diagonally); they can be the same or different shapes.
Kapama Sunglasses is a logic puzzle. A rectangular or square grid contains lines ("bridges") in some cells. The goal is to blacken some cells to create pairs of figures (twin shapes). Twin shapes ("lenses") are symmetrical with respect to a bridge. Two lenses may not share an edge. Cells with bridges can not be blacken. Numbers outside the grid show the number of black cells in a corresponding row or column.
マカロ(Makaro) マサ口(Masakuchi) is a logic puzzle published by Nikoli. A rectangular or square grid is divided into regions. Each region must be filled with each of the digits from 1 to the number of cells in the region. When two numbers are orthogonally adjacent across a region boundary, the numbers must be different. The grid may contain black cells with arrows and numbers: the arrow points at the greatest number among the four cells around (up, under, left, right) the black cell. The number in the black cell shows the difference between the greatest number and the second highest number in all orthogonally adjacent cells around the black cell.
|











![Tapa [Line]](../data/tapa1.gif)

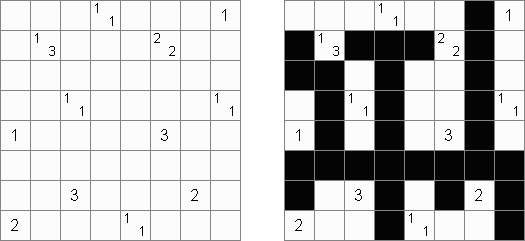

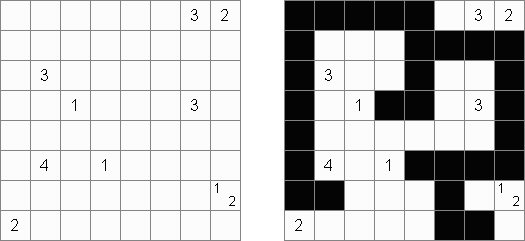
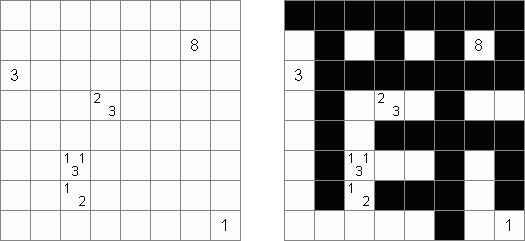

![Tapa [Diagonal Neighbors]](../data/tapa11.gif)